Type II error is mainly caused by the statistical power of a test being low. A Type II error will occur if the statistical test is not powerful enough. The size of the sample can also lead to a Type I error because the outcome of the test will be affected.
What affects a type 2 error?
A Type II error is when we fail to reject a false null hypothesis. Higher values of α make it easier to reject the null hypothesis, so choosing higher values for α can reduce the probability of a Type II error.
What causes type I and type II errors?
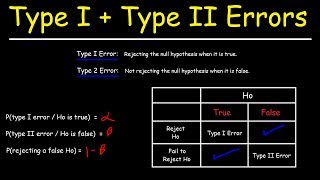
A type I error (false-positive) occurs if an investigator rejects a null hypothesis that is actually true in the population; a type II error (false-negative) occurs if the investigator fails to reject a null hypothesis that is actually false in the population.
When can a type 2 error occur?
A type II error occurs when a false null hypothesis is accepted, also known as a false negative. This error rejects the alternative hypothesis, even though it is not a chance occurence.
How do you know if you made a type 2 error?
When we fail to reject the null hypothesis there are also two possibilities. If the null hypothesis is really true, and there is not a difference in the population, then we made the correct decision. If there is a difference in the population, and we failed to reject it, then we made a Type II error.
How do you determine Type 1 and type 2 errors?
A type 1 error occurs when you wrongly reject the null hypothesis (i.e. you think you found a significant effect when there really isn’t one). A type 2 error occurs when you wrongly fail to reject the null hypothesis (i.e. you miss a significant effect that is really there).
What is Type I and type II error give examples?
In statistical hypothesis testing, a type I error is the mistaken rejection of an actually true null hypothesis (also known as a “false positive” finding or conclusion; example: “an innocent person is convicted”), while a type II error is the failure to reject a null hypothesis that is actually false (also known as a ” …
What causes Type I errors to occur?
What causes type 1 errors? Type 1 errors can result from two sources: random chance and improper research techniques. Random chance: no random sample, whether it’s a pre-election poll or an A/B test, can ever perfectly represent the population it intends to describe.
What is type 2 error in statistics?
Type 2 errors happen when you inaccurately assume that no winner has been declared between a control version and a variation although there actually is a winner. In more statistically accurate terms, type 2 errors happen when the null hypothesis is false and you subsequently fail to reject it.
What decreases a Type 2 error?
While it is impossible to completely avoid type 2 errors, it is possible to reduce the chance that they will occur by increasing your sample size. This means running an experiment for longer and gathering more data to help you make the correct decision with your test results.
Does sample size affect Type 2 error?
Type II errors are more likely to occur when sample sizes are too small, the true difference or effect is small and variability is large. The probability of a type II error occurring can be calculated or pre-defined and is denoted as β.
Does Type 2 error depend on data?
Probability of Type II errors In turn, this value depends on the data used to compute the statistic. But the data is random. Thus, before the data is observed, the test statistic can be regarded as a random variable.
How does power affect Type 2 error?
Simply put, power is the probability of not making a Type II error, according to Neil Weiss in Introductory Statistics. Mathematically, power is 1 – beta. The power of a hypothesis test is between 0 and 1; if the power is close to 1, the hypothesis test is very good at detecting a false null hypothesis.
How does sample size affect type 2 error?
Type II errors are more likely to occur when sample sizes are too small, the true difference or effect is small and variability is large. The probability of a type II error occurring can be calculated or pre-defined and is denoted as β.
How can type 2 error be prevented?
How to avoid type 2 errors. While it is impossible to completely avoid type 2 errors, it is possible to reduce the chance that they will occur by increasing your sample size. This means running an experiment for longer and gathering more data to help you make the correct decision with your test results.
What is Type 2 error in simple words?
What Is a Type II Error? A type II error is a statistical term used within the context of hypothesis testing that describes the error that occurs when one fails to reject a null hypothesis that is actually false. A type II error produces a false negative, also known as an error of omission.
What is Type 1 Type 2 Type 3 error?
Type I error: “rejecting the null hypothesis when it is true”. Type II error: “failing to reject the null hypothesis when it is false”. Type III error: “correctly rejecting the null hypothesis for the wrong reason”.
What are type I and type II errors quizlet?
Type I error. False positive: rejecting the null hypothesis when the null hypothesis is true. Type II error. False negative: fail to reject/ accept the null hypothesis when the null hypothesis is false.
What is the probability of making a Type 2 error?
What is the probability of a Type II error? Step 1: Based on the above question, Power = 0.85. This means that the probability of correctly rejecting the null hypothesis is 0.85 or 85%.
Which of the following gives the probability of making a type II error?
The probability of making a type II error is called Beta (β), and this is related to the power of the statistical test (power = 1- β). You can decrease your risk of committing a type II error by ensuring your test has enough power.
What is Type 2 error in statistics?
Type 2 errors happen when you inaccurately assume that no winner has been declared between a control version and a variation although there actually is a winner. In more statistically accurate terms, type 2 errors happen when the null hypothesis is false and you subsequently fail to reject it.
Which of the following gives the probability of making a type II error?
The probability of making a type II error is called Beta (β), and this is related to the power of the statistical test (power = 1- β). You can decrease your risk of committing a type II error by ensuring your test has enough power.