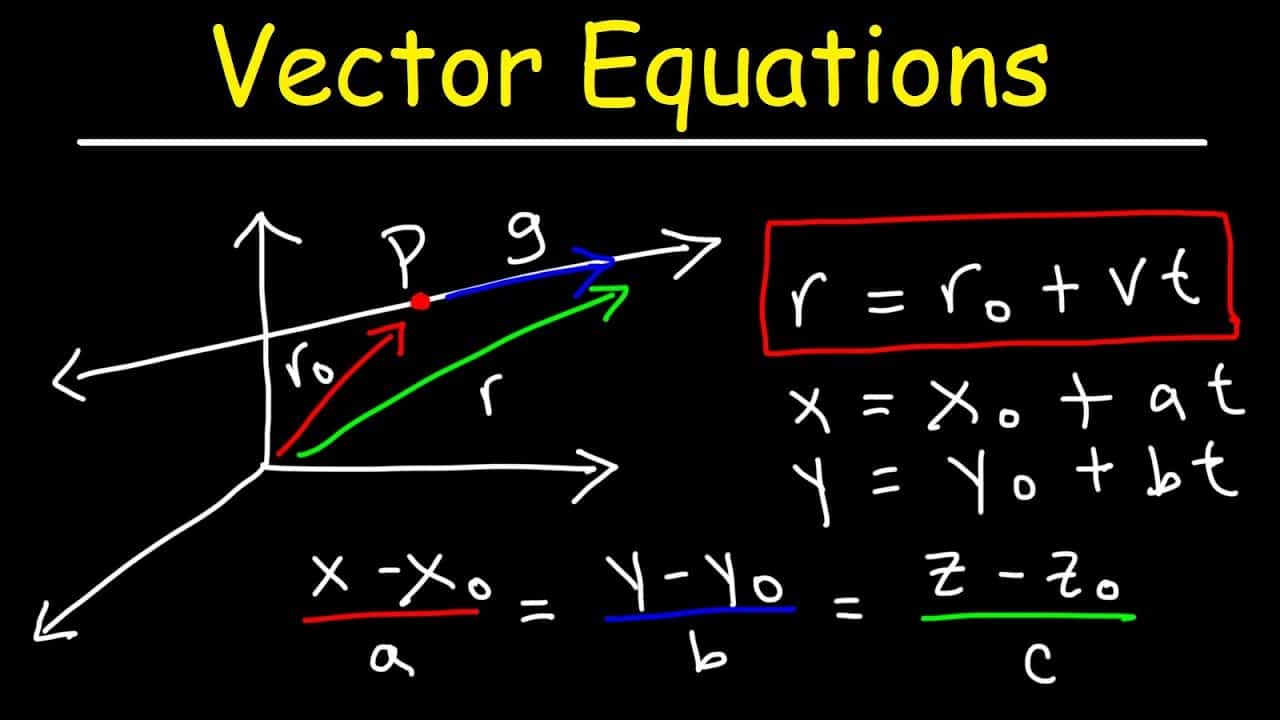
A vector equation is an equation involving a linear combination of vectors with possibly unknown coefficients. Asking whether or not a vector equation has a solution is the same as asking if a given vector is a linear combination of some other given vectors.
What is a vector equation example?
The vector equation of a line passing through a point →a , and parallel to a vector line. Therefore the vector equation of a line passing through a point and is parallel to another vector is →r=3^i+5^j−2^k+λ(5^i+^j+4^k) r → = 3 i ^ + 5 j ^ − 2 k ^ + λ ( 5 i ^ + j ^ + 4 k ^ ) .
What is a vector equation of a plane?
Definition: Vector Form of the Equation of a Plane The vector form of the equation of a plane in ℝ is ⃑ 𝑛 ⋅ ⃑ 𝑟 = ⃑ 𝑛 ⋅ ⃑ 𝑟 , where ⃑ 𝑟 is the position vector of any point that lies on the plane and ⃑ 𝑛 is a normal vector that is perpendicular to the plane or any vector parallel to the plane.
What is a vector equation example?
The vector equation of a line passing through a point →a , and parallel to a vector line. Therefore the vector equation of a line passing through a point and is parallel to another vector is →r=3^i+5^j−2^k+λ(5^i+^j+4^k) r → = 3 i ^ + 5 j ^ − 2 k ^ + λ ( 5 i ^ + j ^ + 4 k ^ ) .
How do you find a vector in math?
Given a position vector →v=⟨a,b⟩,the magnitude is found by |v|=√a2+b2. The direction is equal to the angle formed with the x-axis, or with the y-axis, depending on the application. For a position vector, the direction is found by tanθ=(ba)⇒θ=tan−1(ba), as illustrated in Figure 8.8. 6.
Can two vectors form a plane?
Since a vector has magnitude and direction but no fixed position, vectors alone can never uniquely define a plane. In general, two vectors will define a family of planes that are all parallel to one another; we could call it a stack of planes. But these two vectors are parallel.
What is a scalar equation?
An equation that is not concerned with direction of the quantities is a scalar equation.
How do you write a vector equation from a matrix?
A matrix equation is an equation of the form Ax = b , where A is an m × n matrix, b is a vector in R m , and x is a vector whose coefficients x 1 , x 2 ,…, x n are unknown.
What is a vector equation example?
The vector equation of a line passing through a point →a , and parallel to a vector line. Therefore the vector equation of a line passing through a point and is parallel to another vector is →r=3^i+5^j−2^k+λ(5^i+^j+4^k) r → = 3 i ^ + 5 j ^ − 2 k ^ + λ ( 5 i ^ + j ^ + 4 k ^ ) .
What is a vector equation of a plane?
Definition: Vector Form of the Equation of a Plane The vector form of the equation of a plane in ℝ is ⃑ 𝑛 ⋅ ⃑ 𝑟 = ⃑ 𝑛 ⋅ ⃑ 𝑟 , where ⃑ 𝑟 is the position vector of any point that lies on the plane and ⃑ 𝑛 is a normal vector that is perpendicular to the plane or any vector parallel to the plane.
What does vector mean in maths?
vector, in mathematics, a quantity that has both magnitude and direction but not position. Examples of such quantities are velocity and acceleration.
What does a vector look like?
Definition of a vector. A vector is an object that has both a magnitude and a direction. Geometrically, we can picture a vector as a directed line segment, whose length is the magnitude of the vector and with an arrow indicating the direction. The direction of the vector is from its tail to its head.
How many vectors define a plane?
A plane is a two-dimensional doubly ruled surface spanned by two linearly independent vectors. The generalization of the plane to higher dimensions is called a hyperplane.
What is the difference between a matrix and a vector?
A vector is a list of numbers (can be in a row or column), A matrix is an array of numbers (one or more rows, one or more columns).
What is vector in matrix?
A vector is a matrix with one row or one column. In this chapter, a vector is always a matrix with one column as. [ x1.
How do you find a vector of a matrix?
Matrix-vector product If we let Ax=b, then b is an m×1 column vector. In other words, the number of rows in A (which can be anything) determines the number of rows in the product b. The general formula for a matrix-vector product is Ax=[a11a12… a1na21a22…
What is a vector equation example?
The vector equation of a line passing through a point →a , and parallel to a vector line. Therefore the vector equation of a line passing through a point and is parallel to another vector is →r=3^i+5^j−2^k+λ(5^i+^j+4^k) r → = 3 i ^ + 5 j ^ − 2 k ^ + λ ( 5 i ^ + j ^ + 4 k ^ ) .
What is a vector equation of a plane?
Definition: Vector Form of the Equation of a Plane The vector form of the equation of a plane in ℝ is ⃑ 𝑛 ⋅ ⃑ 𝑟 = ⃑ 𝑛 ⋅ ⃑ 𝑟 , where ⃑ 𝑟 is the position vector of any point that lies on the plane and ⃑ 𝑛 is a normal vector that is perpendicular to the plane or any vector parallel to the plane.
What is the full meaning of vector?
vector, in physics, a quantity that has both magnitude and direction. It is typically represented by an arrow whose direction is the same as that of the quantity and whose length is proportional to the quantity’s magnitude. Although a vector has magnitude and direction, it does not have position.
What is a vector structure?
A vector, in computing, is generally a one-dimensional array, typically storing numbers. Vectors typically have fixed sizes, unlike lists and queues. The vector data structure can be used to represent the mathematical vector used in linear algebra.
Is force a vector?
A force has both magnitude and direction, therefore: Force is a vector quantity; its units are newtons, N.
Why is it called vector?
The term vector comes from engineering/physics. Vectors represent 2 and 3 dimensional lines that have a direction.
What is the vectors equation of a line?
The vectors equation of a line shows us how we can model lines with direction and in three-dimensional space. Through vectors, we’ll have another way to uniquely define a straight line.
How do you find the vector equation if two points are known?
If two points on the line are known, we can find out the line’s vector equation. Similarly, if we know the position vectors of two points op and oq on a line, we can also determine the vector equation of the line by using the vector subtraction method.
What are the different formulas for vectors?
Some of the most important formulas for vectors such as the magnitude, the direction, the unit vector, addition, subtraction, scalar multiplication and cross product are presented. Vector Defined by two Points The components of a vector defined by two points and are given as follows:
Why do we use vector equations in physics?
We use vector equations to find out various physical quantities in 2-D or 3-D, such as velocity, acceleration, momentum, etc. Vector equations give us a diverse and more geometric way of viewing and solving the linear system of equations.